Converse

Converse Statement~ The converse of an if-then statement is the statement formed by switching the hypothesis and the conclusion. It can be true or false.

True Converse Statement~ If you see the ocean, then you are at the beach. If you are at the beach, then you see the ocean.

False Converse Statement~ If you are eating ice-cream, then you are at Chickfila. If you are at Chickfila, then you are eating ice-cream.

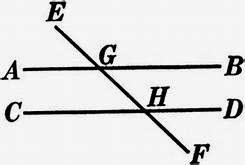
Parallel vs. Non-Parallel

 A Line Crosses A Pair of Parallel Lines

 

    If a set of 2 parallel lines, line l and line m, are crossed or cut by another line, line n, we say "a set of parallel lines are cut by a transversal."

    Each of the parallel lines cut by the transversal has 4 angles surrounding the intersection.

 

    These are matched in measure and position with a counterpart at the other parallel line.

 

    At each of the parallel lines, there are two pairs of vertical angles. Each angle in the pair is congruent to the other angle in the pair.

 

 1  4, angle 1 is congruent to angle 4.

 2  3, angle 2 is congruent to angle 3.

 

 5  8, angle 5 is congruent to angle 8.

 6  7, angle 6 is congruent to angle 7.

                                                 Parallel Lines Cut by a Transversal 

Non-Parallel Lines Cut by a Transversal

Transversal

A line that cuts across a set of lines or the side of a plane figure. Transversals often cut across parallel lines.

Properties of Transversals

                                                       Two Lines Cut by a Transversal

Transversal~ is a line that intersects two or more coplanar lines at different points
Corresponding Angles~ two angles that occupy corresponding positions
Alternate Interior Angles~ two angles that lie between the two lines on the opposite sides of the transversal
Alternate Exterior Angles~ two angles that lie outside the two lines on the opposite sides of the transversal
Same-Side Interior Angles~ two angles that lie between the two lines on the same side of the transversal

Advertising Project

Inductive Reasoning is reasoning in which the premises seek to apply strong evidence for the truth of the conclusion. Deductive Reasoning is reasoning the process of reasoning from one or more statements to reach a logically certain conclusion.


https://www.youtube.com/watch?v=fG3_kC5Gxv0

Overview~ In this clip, they are using inductive reasoning to prove to the viewers that faster is better. They use little kids in this advertisement to show that faster is better in all different situations. 

If faster is better, then slower is worse. 

Reason for Reason- Inductive reasoning seeks to apply strong evidence for the subject being proven. The people who make advertisements every day, seek for people to remember their clips, and buy their products. 

Personal Relation~ This relates to us everyday, because we are a generation of inductive reasoning. We might have a question in class, and give proof to back up our statement. We also might want to campaign for something, and to get people to vote for us, we show them with proof how we can be a great leader.